n, the so-called principal quantum number. It is 1 for the lowest-energy state (the “ground state”) and ranges upward through all the positive integers as the electron ranges farther and farther from the nucleus, and as the binding energy diminishes. (See the figure in Essay Q13.)
ℓ, the orbital angular momentum quantum number. It can take integer values from zero to n – 1. Thus for n = 1, it can have only the value zero; the electron in its lowest-energy state has no orbital angular momentum. For n = 4, ℓ can have the values 0, 1, 2, or 3, corresponding roughly to different eccentricities of elliptical orbits, from a straight in-and-out “orbit” for ℓ = 0 to a circular orbit for ℓ = 3.
mℓ, the orbital orientation quantum number. It ranges from – ℓ to + ℓ in integer steps, and tells the orientation in space of the orbital angular momentum vector relative to a chosen axis.
ms, the spin orientation quantum number. It can take the values – ½ and + ½, and tells the orientation in space of the electron’s spin vector relative to a chosen axis. (Spin itself does not require a quantum number because it has only one value.)
At first thought, there seems to be no similarity at all between a quantum wave spread over the interior volume of an atom and a speck of matter (an electron) following an orbital track within the atom. Yet there is an interesting correspondence between these quantum and classical descriptions, and it becomes more pronounced as the quantum number n gets larger. This correspondence is illustrated in the figure below, which shows the cross sections of electron waves across a diameter from one side of a hydrogen atom to the opposite side (radial in and radial out) for four states in the hydrogen atom: The graphs are labeled by the principal quantum number n, which determines the energy, and the orbital angular quantum number ℓ. (a) The “ground state,” n = 1, ℓ = 0. This is the lowest energy state, and has no angular momentum. (b) The state n = 4, ℓ = 0. This is the fourth state up the energy ladder, also with no angular momentum. (c) The state n = 4, ℓ = 3. This is a state with the same energy as in example (b) but corresponding to classical circular motion instead of linear in-and-out motion. (d) The state n = 20, ℓ = 19, corresponding to a very large circular orbit. Radial scales are not the same in the different graphs.
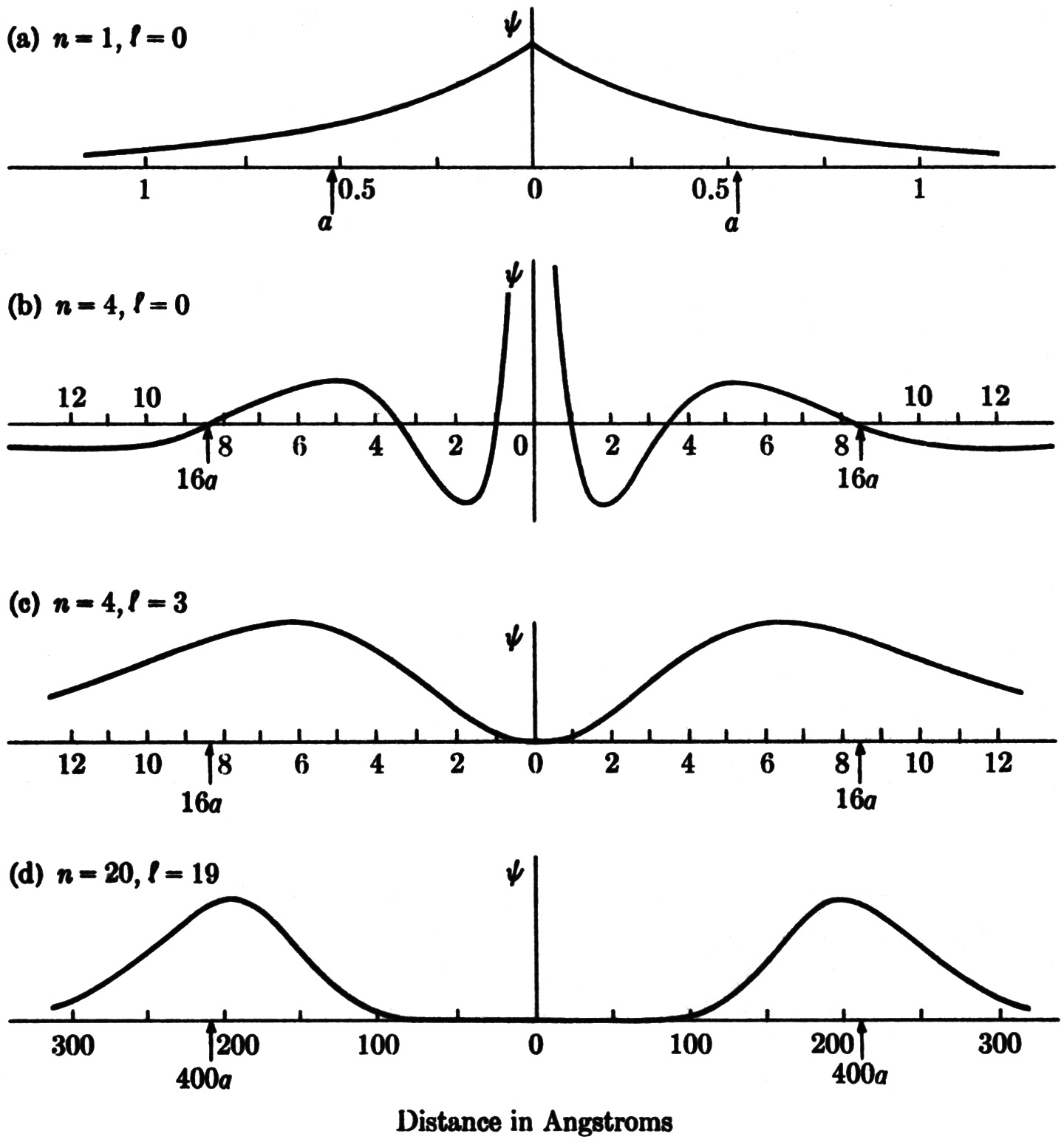 (a) The most featureless wave is that of the ground state (n = 1, ℓ = 0). Lacking any angular momentum, this state also lacks a preferred direction in space, and is characterized by a spherically symmetric wave. In the radial direction the wave behaves in the simplest possible manner, rising to a single maximum at the center of the atom, then falling again.
(a) The most featureless wave is that of the ground state (n = 1, ℓ = 0). Lacking any angular momentum, this state also lacks a preferred direction in space, and is characterized by a spherically symmetric wave. In the radial direction the wave behaves in the simplest possible manner, rising to a single maximum at the center of the atom, then falling again.
(b) For the higher energy state with n = 4 and ℓ = 0, the absence of angular momentum is again reflected in the spherical symmetry of the wave. To visualize the classical analogue of this state of motion, think of a long narrow ellipse squeezed to become a straight line. In the quantum wave, this to-and-fro motion shows itself in the radial oscillation of the wave. Having a greater distance to cover at n = 4 than at n = 1, the electron wave undergoes a number of cycles of oscillation from one side of the atom to the other.
(c) At the same energy level (n = 4), the wave function of a state of higher angular momentum (ℓ = 3) also shows oscillatory behavior, but directed around the nucleus in orbital fashion rather than toward and away from the nucleus. This wave, representing one of Bohr’s circular orbits, is concentrated in a doughnut around the nucleus.
(d) As quantum numbers increase in magnitude, and the granular effects of quantum mechanics become less significant, the wave concept begins to resemble closely the classical-orbit concept. In this diagram the quantum wave has narrowed into a shell with a large hollow center. The wave oscillates as it follows around a circular Bohr orbit, closing upon itself with constructive interference in much the manner visualized by de Broglie. The gradual transition from quantum to classical behavior is one of the beautiful aspects of the submicroscopic world.
Note in each graph above that the radial distance an2 is flagged, where a is the “Bohr radius.” For the “circular orbits,” these distances, a, 16a, and 400a, do indeed indicate where the electron is likely to be found.
As a further aid to visualizing the correspondence between classical orbits and quantum waves, I show below pictorial representations of the probability density for each of these four quantum states.
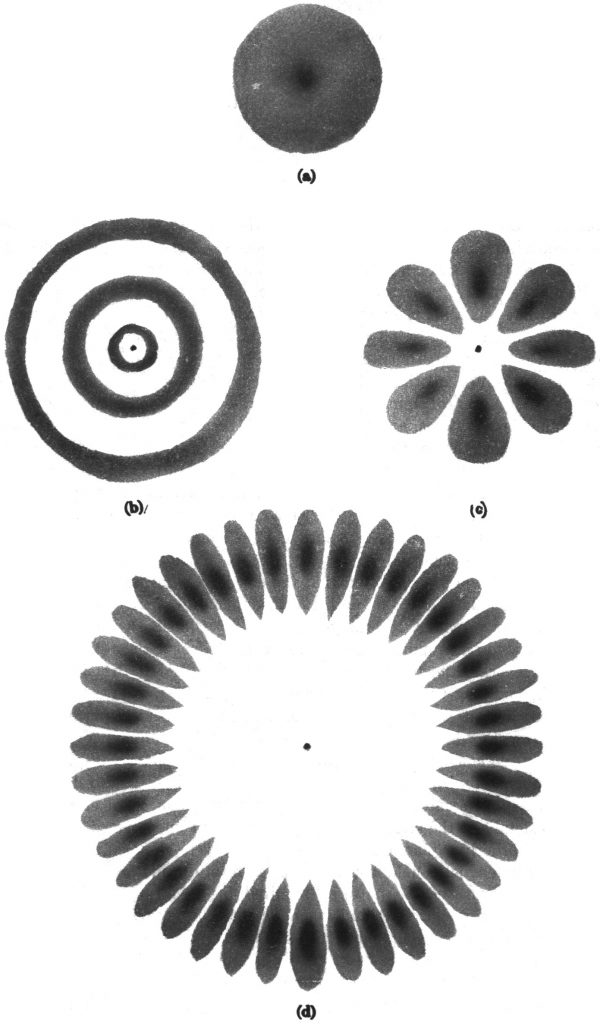 (a) The “blob” represents an electron oscillating in and out along a radial direction, with all directions equally likely. From one side of the atom to the other can be considered half a wavelength (the wave amplitude rises and falls just once).
(a) The “blob” represents an electron oscillating in and out along a radial direction, with all directions equally likely. From one side of the atom to the other can be considered half a wavelength (the wave amplitude rises and falls just once).
(b) Just as in diagram (a), the electron’s motion is in and out through the nucleus (again no orbital motion), but this time with several wavelengths from one side of the atom to the other. The “rings” in the drawing are points of maximum probability to find the electron.
(c) This state has the same energy as the one depicted in diagram (b) but with maximum orbital angular momentum. The electron is circling around the nucleus instead of moving radially in and out. The eight “lobes” are regions of greatest probability to find the electron.
(d) This “circular” state at n = 20 does indeed look like a de Broglie wave. The quantum/classical correspondence is evident.
